![\includegraphics[width=0.9\textwidth,clip, clip]{SelfE.eps}](img392.png) |
In the SCBA the electron self-energy is obtained by summing over all non-crossing diagrams. In this approximation the calculation of the self-energy can be reduced to the following set of equations:
![\includegraphics[width=0.9\textwidth,clip, clip]{SelfE.eps}](img392.png) |
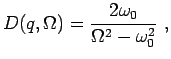 |
(4.19) |
and
![\includegraphics[width=0.9\textwidth,clip, clip]{QMCvsSCA_lamb.eps}](img404.png) |
The neglect of vertex corrections in the SCBA
results in a failure of this approximation in the intermediate and strong coupling regime.
This is apparent in Fig. 4.2 where a comparison of SCBA and exact QMC
results for the H model energy and quasiparticle weight at ![]() is shown.
In fact the polaron properties at
is shown.
In fact the polaron properties at ![]() are better approximated in a
regular Rayleigh-Schrodinger (RS) perturbation theory than in SCBA (35). However,
at large
are better approximated in a
regular Rayleigh-Schrodinger (RS) perturbation theory than in SCBA (35). However,
at large ![]() where the polaron energy approaches the phonon frequency,
the RS perturbation theory fails and the SCBA which
considers configurations involving a large number of phonons
provides good results. In order to show that, we compare
the SCBA results with the exact ones obtained by using
the diagrammatic quantum Monte Carlo technique(36,37) in Fig. 4.3.
As can be seen in Fig. 4.3 -a, the dispersion becomes flat
when the polaron energy gets close to the the phonon frequency
where the polaron energy approaches the phonon frequency,
the RS perturbation theory fails and the SCBA which
considers configurations involving a large number of phonons
provides good results. In order to show that, we compare
the SCBA results with the exact ones obtained by using
the diagrammatic quantum Monte Carlo technique(36,37) in Fig. 4.3.
As can be seen in Fig. 4.3 -a, the dispersion becomes flat
when the polaron energy gets close to the the phonon frequency
![]() ,
, ![]() being the bottom of the polaronic band.
The flattening is accompanied by a strong reduction of the quasiparticle weight,
as shown in Fig. 4.3 -b.
The SCBA slightly underestimates the energy corrections in the flat
dispersion region but otherwise captures all these features successfully.
being the bottom of the polaronic band.
The flattening is accompanied by a strong reduction of the quasiparticle weight,
as shown in Fig. 4.3 -b.
The SCBA slightly underestimates the energy corrections in the flat
dispersion region but otherwise captures all these features successfully.
![\includegraphics[width=0.9\textwidth,clip, clip]{qmcscba.eps}](img406.png) |
The H polaron physics at small coupling
has been investigated with different techniques
for many years and is rather well understood now. The polaron
character changes from almost free electron at
the bottom of the band to a one phonon plus one electron
state in the flat dispersion region where almost all the momentum is carried by the phonon.
The states below the one phonon threshold
![]() are bound states(11),
characterized by delta peaks in the spectral representation. Above it, there is a continuum
of states and the electron self-energy acquires a finite imaginary part.
However, at large energies relative to
are bound states(11),
characterized by delta peaks in the spectral representation. Above it, there is a continuum
of states and the electron self-energy acquires a finite imaginary part.
However, at large energies relative to ![]() the self-energy's imaginary part is small and the spectral
representation is characterized by sharp peaks with a dispersion close to
the free electron one. In a photoemission experiment the dispersion
of the high intensity peak would exhibit something resembling
a kink, although a small gap at the phonon energy
and sudden onset of broadening appears at the same time.
The spectral function at momenta corresponding to energies
well below the phonon frequency will consist of a sharp peak followed
by a broad satellite, such as it is observed in surface studies of
the self-energy's imaginary part is small and the spectral
representation is characterized by sharp peaks with a dispersion close to
the free electron one. In a photoemission experiment the dispersion
of the high intensity peak would exhibit something resembling
a kink, although a small gap at the phonon energy
and sudden onset of broadening appears at the same time.
The spectral function at momenta corresponding to energies
well below the phonon frequency will consist of a sharp peak followed
by a broad satellite, such as it is observed in surface studies of ![]() (19) for instance.
This can be seen in Fig. 4.3 c) where
a false color plot of spectral intensity obtained with SCBA is shown (see also Fig. 4.8 -a).
The physics discussed in this paragraph (i.e. the dispersion flattening and the gap in the polaron
spectrum) is presumably true even for very small electron-phonon couplings.
However, for such small couplings these
features are too small to be captured experimentally or even
numerically.
(19) for instance.
This can be seen in Fig. 4.3 c) where
a false color plot of spectral intensity obtained with SCBA is shown (see also Fig. 4.8 -a).
The physics discussed in this paragraph (i.e. the dispersion flattening and the gap in the polaron
spectrum) is presumably true even for very small electron-phonon couplings.
However, for such small couplings these
features are too small to be captured experimentally or even
numerically.