- ... interpolation 3.1
- We omit the additional
coarse graining of the self-energy in the original work. This is
deemed appropriate since the interpolated small cluster self-energy
wont recoup the lattice self-energy and hence a coarse graining to a
cluster is merit-less.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... 3.2
- The DCA inherently has a lesser
sign problem within the QMC compared to finite size
approaches (2) and in the combination with a smaller cluster
size results in a significantly larger average sign.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... (B) 4.1
- The
breathing mode actually can be properly defined only at the zone
corner in 2D and at
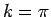 in 1D. However in this chapter we extend
this nomenclature for all the
in 1D. However in this chapter we extend
this nomenclature for all the 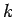 points, even if this is not entirely
correct.
points, even if this is not entirely
correct.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... energy 4.2
- We set the lattice constant equal to
unity.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... dispersion 4.3
- DOS for the
linear dispersion is constant
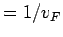 while for a 1D tight-binding
dispersion it is infinite at
while for a 1D tight-binding
dispersion it is infinite at 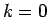 .
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... quasiparticle 4.4
- The physics at energies
close to
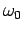 relative to Fermi surface is presumably not influenced much by the electron
hole creation at the Fermi surface.
relative to Fermi surface is presumably not influenced much by the electron
hole creation at the Fermi surface.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.