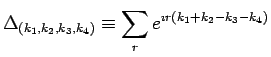 |
(3.2) |
The DCA is a systematic quantum cluster theory that maps the lattice problem onto a self-consistently embedded cluster problem. It is an extension of the dynamical mean-field theory (DMFT)(6,5) which systematically incorporates non-local correlations. In the limit when the cluster size is one (i.e. single site), it recovers the purely local DMFT solution, systematically incorporates non-local corrections as the cluster size increases, and finally becomes exact when the cluster size equals the size of the lattice.
The respective approximations for the DMFT and DCA may be derived by approximating the Laue function which describes momentum conservation at the vertices of the irreducible diagrams:
In the DMFT, the Laue function is
approximated with
![]() for all combinations
of
for all combinations
of ![]() ,
, ![]() ,
, ![]() and
and ![]() . In doing so, all electron
propagators in the self-energy diagrams may be averaged over the first
Brillouin Zone (BZ); thus relinquishing any momentum dependence of the
self-energy. Hence, the DMFT lattice Green's function contains local
correlations of the system but is unable to capture non-local
correlations. The DCA sets out to systematically include these
non-local contributions. This is accomplished by partially restoring
momentum conservation of the irreducible vertices. We divide the BZ
into
. In doing so, all electron
propagators in the self-energy diagrams may be averaged over the first
Brillouin Zone (BZ); thus relinquishing any momentum dependence of the
self-energy. Hence, the DMFT lattice Green's function contains local
correlations of the system but is unable to capture non-local
correlations. The DCA sets out to systematically include these
non-local contributions. This is accomplished by partially restoring
momentum conservation of the irreducible vertices. We divide the BZ
into ![]() identical discrete sub-cells as illustrated in
Fig. 3.1. The center of each cell is labeled by
identical discrete sub-cells as illustrated in
Fig. 3.1. The center of each cell is labeled by ![]() , and the
surrounding points by
, and the
surrounding points by ![]() , so that any arbitrary
, so that any arbitrary
![]() . In the DCA, this partial momentum conservation is
expressed by the Laue function:
. In the DCA, this partial momentum conservation is
expressed by the Laue function:
| (3.4) |
![\includegraphics[width=0.9\textwidth,clip, clip]{DCA.eps}](img236.png) |
Therefore, all propagators may freely be summed over
intra-cell momenta ![]() , yielding the coarse grained Green's
function
, yielding the coarse grained Green's
function
In so doing, only momentum conservation of magnitude
![]() is neglected, while larger inter-cell transfers are
preserved. The resulting self-energy diagrams are now those of a
finite cluster of size
is neglected, while larger inter-cell transfers are
preserved. The resulting self-energy diagrams are now those of a
finite cluster of size ![]() where each lattice propagator has been
replaced by its coarse-grained analog, and the remaining cluster
problem is defined by
where each lattice propagator has been
replaced by its coarse-grained analog, and the remaining cluster
problem is defined by
![]() . We can write for
the DCA lattice Green's function
. We can write for
the DCA lattice Green's function
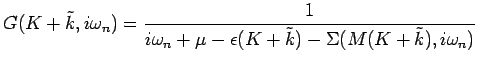 |
(3.6) |
where ![]() is a function which maps momentum
is a function which maps momentum ![]() residing
in a certain sub-cell of the BZ to its cluster momentum
residing
in a certain sub-cell of the BZ to its cluster momentum ![]() and the
lattice self-energy is approximated by that of the cluster problem.
and the
lattice self-energy is approximated by that of the cluster problem.
The remaining embedded cluster problem must be solved
with a self-consistency requirement that the
Green's function calculated on the cluster
![]() . Fig. 3.2 depicts the corresponding DCA
algorithm: Starting with an initial guess for the self-energy, we
construct the coarse-grained Green's function
. Fig. 3.2 depicts the corresponding DCA
algorithm: Starting with an initial guess for the self-energy, we
construct the coarse-grained Green's function
![]() from the
corresponding lattice
from the
corresponding lattice ![]() (see Eq. 3.5). In the next step, we
utilize one of the many available cluster solvers to determine the
cluster self-energy. This is the numerically most involved step and a
variety of numerical techniques may be applied. At this point, we use
the new estimate for the self-energy to re-initialize the
self-consistency loop. It is important to notice that in this
procedure only the irreducible lattice quantities are approximated by
their cluster equivalent - i.e. the self-energy.
(see Eq. 3.5). In the next step, we
utilize one of the many available cluster solvers to determine the
cluster self-energy. This is the numerically most involved step and a
variety of numerical techniques may be applied. At this point, we use
the new estimate for the self-energy to re-initialize the
self-consistency loop. It is important to notice that in this
procedure only the irreducible lattice quantities are approximated by
their cluster equivalent - i.e. the self-energy.
The DCA has been successfully implemented with a variety of cluster solvers of which some are exact but limited in cluster size, while others are applicable up to larger length-scales but involve varying degrees of approximation. Some of the cluster solvers which have been used in conjunction with the DCA include the non-crossing approximation (NCA)(7), the fluctuation exchange approximation (FLEX)(3,8) and the Quantum Monte Carlo (QMC)(2) method. While NCA and FLEX involve various levels of approximations, QMC is of special interest since it provides an essentially numerically exact solution to the problem. Although the QMC constitutes a precise cluster solver, it becomes prohibitively expensive for large clusters. The range of applicability of exact calculations is thus restricted to relatively short length-scales. However, various properties of strongly-correlated systems are not accounted for (e.g. Mermin-Wagner theorem(9)) due to the absence of long-ranged fluctuations in these solutions.