1.2.1 The Kondo Model

Historically, the Kondo model is the first of the common models in the study of strongly correlated systems (A detailed review of the Kondo problem can be found in (9)). It successfully addressed the experimentally observed non-zero low-temperature minimum found in the resistivity $\rho(T)$ of some metals with magnetic impurities. Jun Kondo, by considering higher order perturbation theory, showed that spin-flip scattering of magnetic impurities has an additional logarithmic diverging contribution to the resistivity proportional to the inverse temperature:

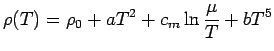$$\rho(T)=\rho_0+aT^2+c_m \ln \frac {\mu}{T}+bT^5$$ (1.1)

The theoretically expected divergence as $T\rightarrow 0$ was re-conciliated with experimental finite measurements by realizing that a competing mechanism successfully suppressed the divergence: The formation of a anti-ferromagnetic bound state between the conduction electrons and the localized spin of the impurity, effectively shields the impurity thus removing its contribution to the resistivity . The formal Hamiltonian describing the single impurity Kondo model (SIKM) in such systems is given by

$$H_{\rm SIKM} = \sum\limits_{\vec k \sigma}\epsilon^{\phantom{\dag... ...igma^{\phantom{\dagger}}_{\alpha\beta}\,c^{\phantom{\dagger}}_{\vec k'\beta}~~.$$ (1.2)

Figure 1.1: A physical interpretation of the underlying coupling in the Kondo model.

Figure 1.1 depicts a physical interpretation of the Kondo model. A single magnetic impurity (small circle) is embedded in a Fermi-sea. While most of the electrons in the Fermi-sea remain free electrons (first part of the Hamiltonian), some in the vicinity of the impurity will couple to the localized spin with an exchange coupling $J$ (second term in the Hamiltonian). In the high temperature limit (top of fig. 1.1), the local moment remains essentially free, un-influenced by the surrounding Fermi-sea. The physical properties of interest occur as the temperature is decreased. Depending on the nature of the coupling $J$ there are two possible scenarios we encounter. For a Ferromagnetic coupling $J<0$, the free local moment remains and is completely decoupled from the Fermi-sea (see case a.). The more interesting case occurs for an anti-ferromagnetic coupling $J>0$ (see case b.). In this situation, the conduction spins in the vicinity of the local moment form a quasi-bound state with the impurity spin. This results in a quenched local moment and a residual Fermi-sea. What remains is a strong renormalization of the properties of the Fermi-sea and the systems are hence qualified as a strongly coupled electron systems. Despite its simplicity and the relatively well understood physical properties the problem still has not been definitively solved yet.

In chapter 2, a new approach to this old model is presented. The SIKM is solved by a semi-analytical approach based on the flow equation method. The resulting problem is shown to be equivalent to a resonant level model with a non-constant hybridization function providing a simple means for evaluating a wide range of static and dynamic quantities of the Kondo model in the strong-coupling limit. This nontrivial effective hybridization function encodes the quasiparticle interaction in the Kondo limit.